
We have also drawn a center point “C” and line segments from the vertices of the triangle to C (all of these line segments have a length of R, the radius of the circle). Here we have labelled the side lengths of our equilateral triangle as “S”. One of the simplest cases is an equilateral triangle (with side length S) inscribed in a circle (with radius R), which you can see below. We can inscribe a triangle inside of a circle. Let’s start with an equilateral triangle inscribed in a circle. With a little algebra, we can calculate the dimensions (side lengths, perimeter, and area) for each of these polygons, given the radius of the circle they are inscribed in. When we inscribe a regular polygon (such as an equilateral triangle or a square) in a circle, the shape is “as large as possible” while still fitting inside of the circle.
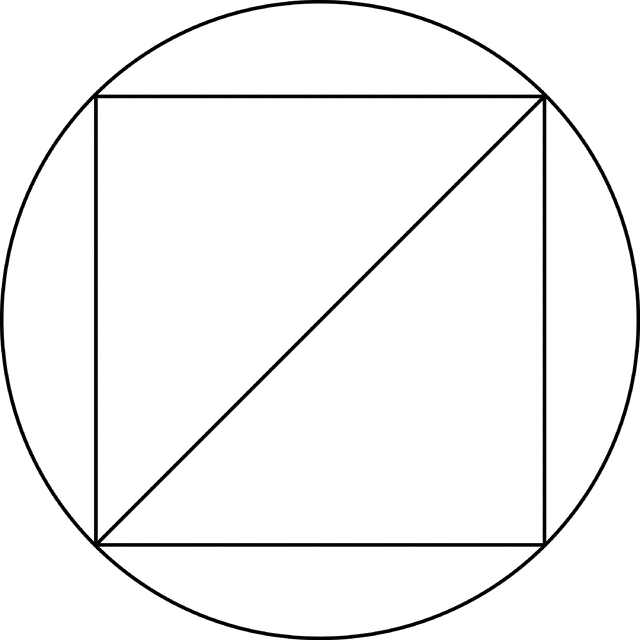
#ISCRIBE A SQUARE INTO A CIRCLE HOW TO#
We’ll also learn how to find the perimeter and area of these shapes. In this article, we’ll talk about inscribed triangles, squares, and other shapes inside of a circle. Of course, we can also inscribe a circle inside of these shapes (triangles, squares, and so forth), which gives us a different result for perimeter and area. We can calculate the perimeter and area for a shape inscribed in a circle.

The vertices (corners) of the shape will intersect the circle on its circumference. We can inscribe lots of different shapes inside of a circle, including triangles, squares, pentagons, and others.Īn inscribed shape inside of a circle is as large as possible while still fitting inside of the circle.


 0 kommentar(er)
0 kommentar(er)
